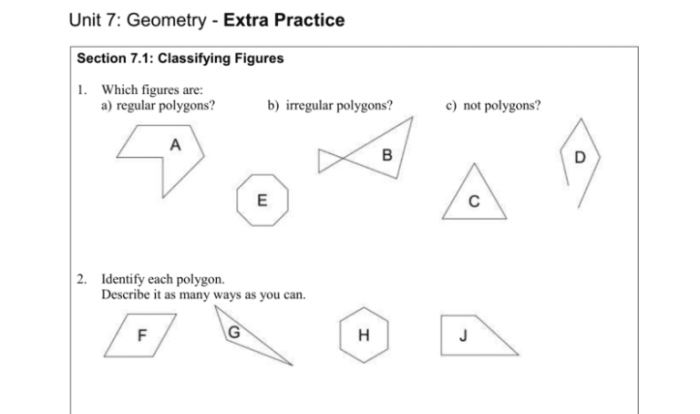
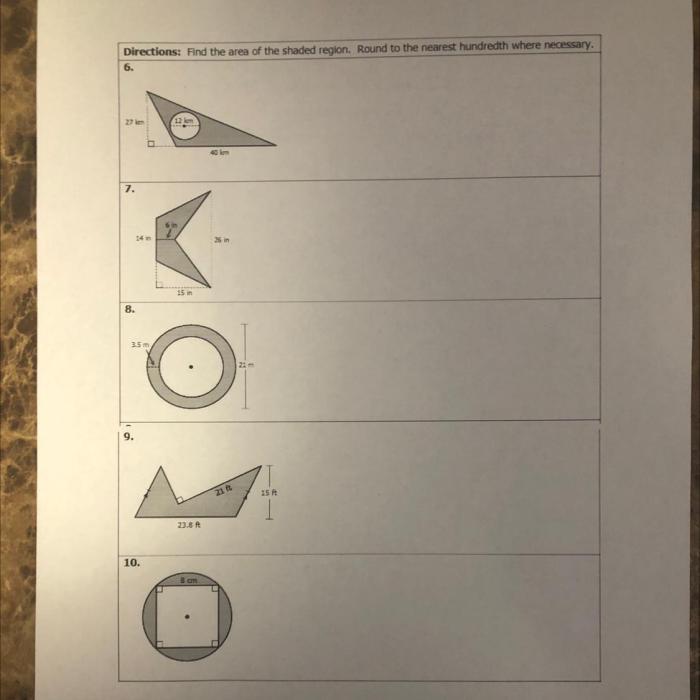
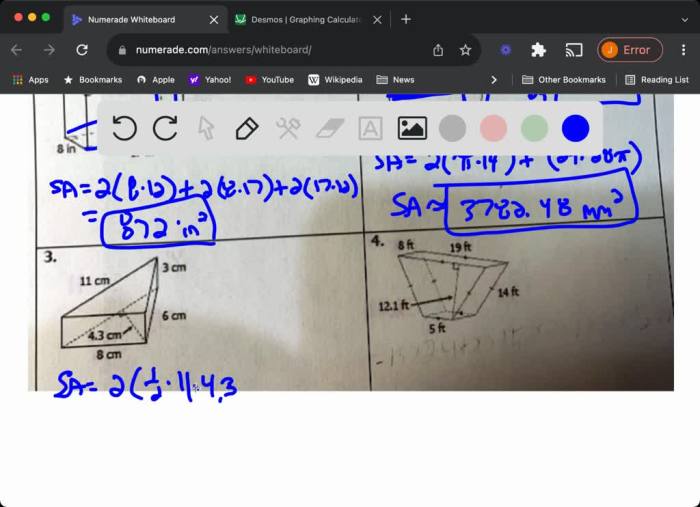
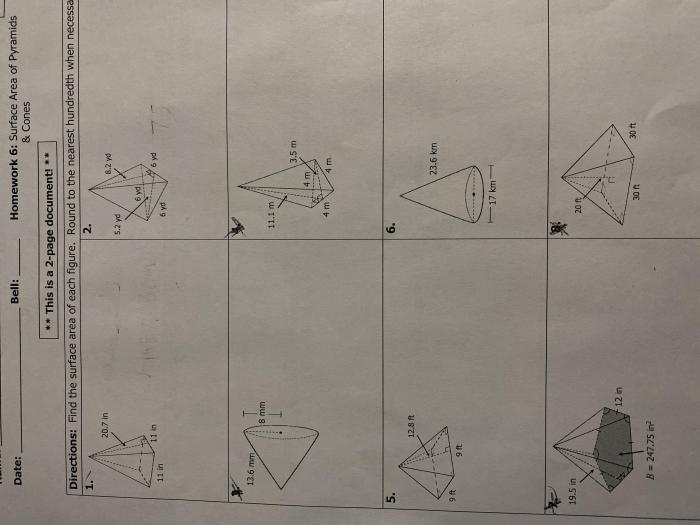
Embark on a captivating journey through Unit 11 Volume and Surface Area Homework 7, a comprehensive exploration of essential geometric concepts that shape our world. This in-depth guide unveils the intricacies of volume and surface area, empowering you with the knowledge to tackle real-world applications with confidence.
Prepare to unravel the mysteries of rectangular prisms, cylinders, cones, and spheres as we delve into the formulas that govern their dimensions. Along the way, discover the significance of these concepts in diverse fields, from engineering to architecture and beyond.
Volume and Surface Area of Rectangular Prisms
Rectangular prisms are three-dimensional shapes with six rectangular faces. The volume of a rectangular prism is the amount of space it occupies, while the surface area is the total area of its surfaces.
To calculate the volume of a rectangular prism, we use the formula:
V = l × w × h
where l is the length, w is the width, and h is the height of the prism.
For example, if a rectangular prism has a length of 5 cm, a width of 3 cm, and a height of 2 cm, its volume would be:
V = 5 cm × 3 cm × 2 cm = 30 cm³
The units used to measure volume are cubic units, such as cubic centimeters (cm³), cubic meters (m³), or cubic inches (in³).
Surface Area of Rectangular Prisms
To calculate the surface area of a rectangular prism, we use the formula:
A = 2(lw + wh + lh)
where l is the length, w is the width, and h is the height of the prism.
For example, if a rectangular prism has a length of 5 cm, a width of 3 cm, and a height of 2 cm, its surface area would be:
A = 2(5 cm × 3 cm + 3 cm × 2 cm + 5 cm × 2 cm) = 62 cm²
The units used to measure surface area are square units, such as square centimeters (cm²), square meters (m²), or square inches (in²).
Volume and Surface Area of Cylinders
Cylinders are three-dimensional shapes with two circular bases and a curved surface. The volume of a cylinder is the amount of space it occupies, while the surface area is the total area of its surfaces.
To calculate the volume of a cylinder, we use the formula:
V = πr²h
where r is the radius of the base and h is the height of the cylinder.
For example, if a cylinder has a radius of 5 cm and a height of 10 cm, its volume would be:
V = π × 5 cm² × 10 cm = 250π cm³ ≈ 785.4 cm³
The units used to measure volume are cubic units, such as cubic centimeters (cm³), cubic meters (m³), or cubic inches (in³).
Surface Area of Cylinders
To calculate the surface area of a cylinder, we use the formula:
A = 2πr(r + h)
where r is the radius of the base and h is the height of the cylinder.
For example, if a cylinder has a radius of 5 cm and a height of 10 cm, its surface area would be:
A = 2π × 5 cm × (5 cm + 10 cm) = 100π cm² ≈ 314.2 cm²
The units used to measure surface area are square units, such as square centimeters (cm²), square meters (m²), or square inches (in²).
Volume and Surface Area of Cones: Unit 11 Volume And Surface Area Homework 7
Cones are three-dimensional shapes with a circular base and a single vertex. The volume of a cone is the amount of space it occupies, while the surface area is the total area of its surfaces.
To calculate the volume of a cone, we use the formula:
V = (1/3)πr²h
where r is the radius of the base and h is the height of the cone.
For example, if a cone has a radius of 5 cm and a height of 10 cm, its volume would be:
V = (1/3)π × 5 cm² × 10 cm = (50/3)π cm³ ≈ 52.36 cm³
The units used to measure volume are cubic units, such as cubic centimeters (cm³), cubic meters (m³), or cubic inches (in³).
Surface Area of Cones
To calculate the surface area of a cone, we use the formula:
A = πr(r + l)
where r is the radius of the base and l is the slant height of the cone.
For example, if a cone has a radius of 5 cm and a slant height of 10 cm, its surface area would be:
A = π × 5 cm × (5 cm + 10 cm) = 75π cm² ≈ 235.62 cm²
The units used to measure surface area are square units, such as square centimeters (cm²), square meters (m²), or square inches (in²).
Volume and Surface Area of Spheres
Spheres are three-dimensional shapes with a perfectly round surface. The volume of a sphere is the amount of space it occupies, while the surface area is the total area of its surface.
To calculate the volume of a sphere, we use the formula:
V = (4/3)πr³
where r is the radius of the sphere.
For example, if a sphere has a radius of 5 cm, its volume would be:
V = (4/3)π × 5 cm³ = (200/3)π cm³ ≈ 261.8 cm³
The units used to measure volume are cubic units, such as cubic centimeters (cm³), cubic meters (m³), or cubic inches (in³).
Surface Area of Spheres, Unit 11 volume and surface area homework 7
To calculate the surface area of a sphere, we use the formula:
A = 4πr²
where r is the radius of the sphere.
For example, if a sphere has a radius of 5 cm, its surface area would be:
A = 4π × 5 cm² = 100π cm² ≈ 314.2 cm²
The units used to measure surface area are square units, such as square centimeters (cm²), square meters (m²), or square inches (in²).
Applications of Volume and Surface Area
Volume and surface area are important concepts in various fields, including engineering, architecture, and design.
- In engineering, volume and surface area are used to calculate the capacity of containers, the strength of structures, and the efficiency of engines.
- In architecture, volume and surface area are used to determine the size of buildings, the amount of materials needed, and the energy efficiency of structures.
- In design, volume and surface area are used to create objects that are both functional and aesthetically pleasing.
Question Bank
What is the formula for calculating the volume of a rectangular prism?
Volume = length × width × height
How do I find the surface area of a cylinder?
Surface Area = 2πr(r + h)
What units are commonly used to measure volume?
Cubic units, such as cubic centimeters (cm³), cubic meters (m³), and cubic feet (ft³)